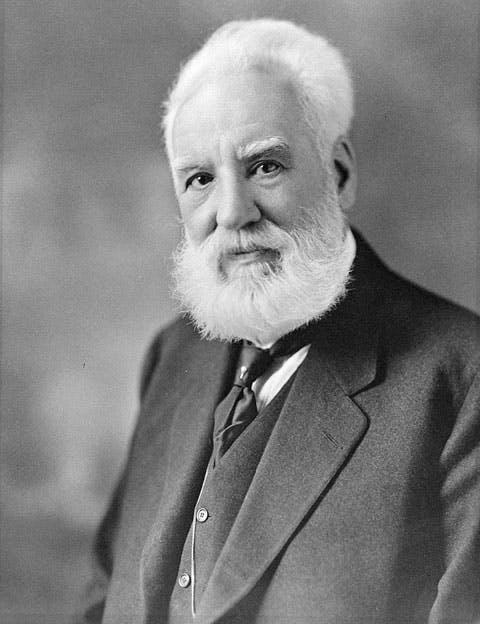As Part 1 introduces, we get decibel using the formula

$$ l = 10 \times log \frac{p_1}{p_2} (dB) $$

I'd blame my poor English ability that I didn't realise what the name decibel really meant for quite a few years. It's called deci-bel so apparently there's a Bel first.

The bel (B) and the smaller decibel (dB) are units of measurement of sound pressure level (SPL) invented by Bell Labs and named after him

from: Wikipedia

That's him! As stated, using deci-, people can make bel smaller, and meanwhile we have to add the \(10 \times \) in the formula, to make bel become decibel.

You might notice that when talking about voltage and current, the formula becomes

$$ 20 \times log \frac{p_1}{p_2} (dB) $$

So why 20 here? I was very confused. I asked around and got this answer: For measuring something like a magnitude, we use \(20 \times \), otherwise for the power stuffs, use \(10 \times \)... Okay then it's time to test my English again: what kind of thing is a magnitude? And what is not? I reckon it's a correct but not so good answer.

Finally I met a guy, he took out a piece of paper and wrote some high school maths on it:

$$ P=U \times I=U \times (U/R) = U^2 / R $$

Yeah that's Ohm's Law, I get it. Then if \(P_r\) and \(U_r\) are the references and \(U\) is the voltage we are measuring, from the original \(10 \times \) formula we have:

\[ 10 \times log (P / P_r) \]

\[ = 10 \times log ((U^2/R)/(U_r^2/R)) \]

\[ = 10 \times log (U/U_r)^2 \]

\[ = 2 \times 10 \times log (U/U_r) = 20 \times log (U/U_r) \]

That's how \(20 \times \) comes up. Same process for the current (Use \(P=I^2 \times R\)). Maths can be scary, but useful.